


















Do you want to contribute by writing guest posts on this blog?
Please contact us and send us a resume of previous articles that you have written.
The Fascinating Journey: From Single Neurons To Neural Fields

Have you ever wondered how our brains, the center of our thoughts and perceptions, function? With billions of neurons working together, the brain is a complex organ that allows us to experience the world around us. But how does it all work? How do single neurons combine to create such intricate neural fields?
In this article, we will dive into the captivating world of mathematical modeling in neuroscience, exploring the journey from single neurons to neural fields. Get ready to unravel the mysteries of the brain and discover the amazing power of mathematical models in understanding its inner workings.
Understanding Neurons: The Building Blocks of the Brain
4 out of 5
| Language | : | English |
| File size | : | 965 KB |
| Text-to-Speech | : | Enabled |
| Screen Reader | : | Supported |
| Enhanced typesetting | : | Enabled |
| Word Wise | : | Enabled |
| Print length | : | 67 pages |
| Lending | : | Enabled |
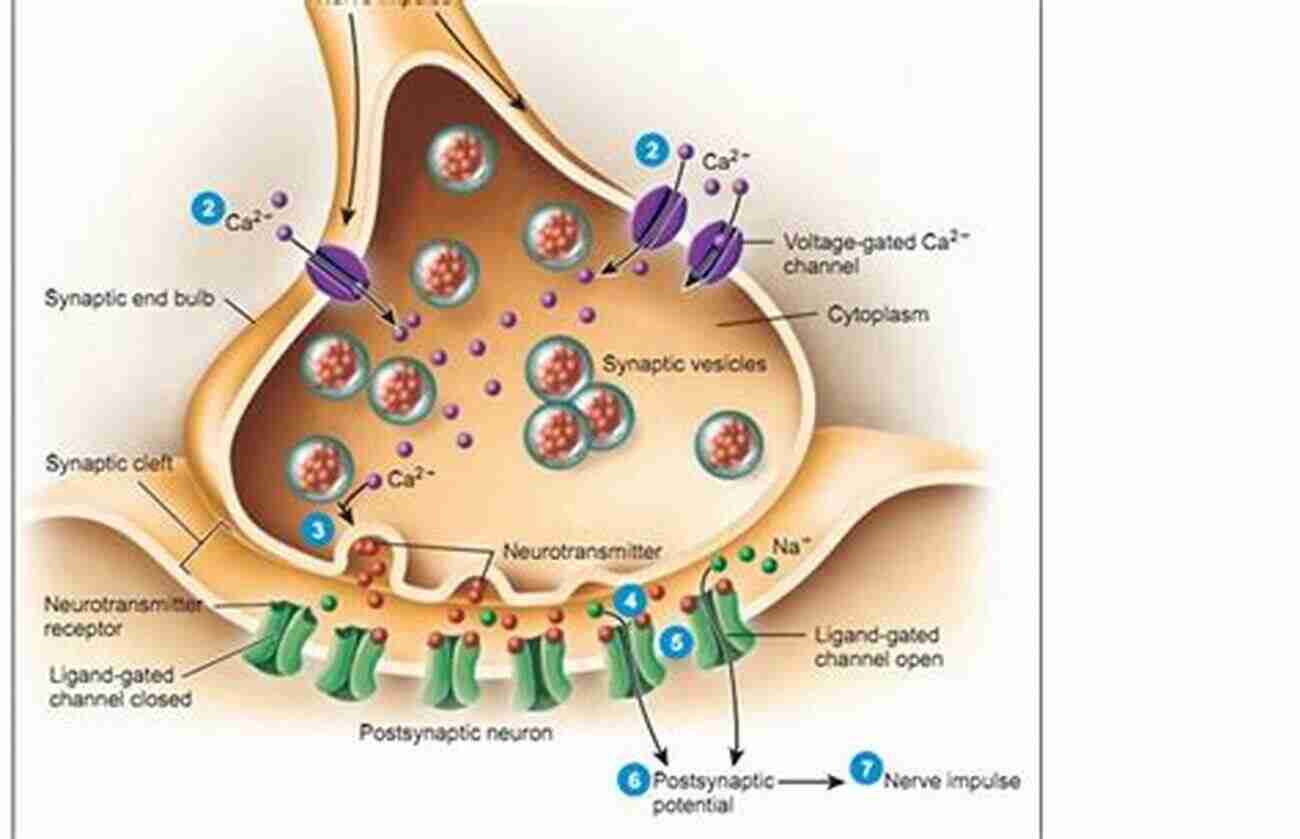
Neurons, often called the "building blocks" of the brain, are specialized cells that transmit information through electrical and chemical signals. Each neuron has a cell body, dendrites (which receive signals),and an axon (which sends signals to other neurons). Neurons communicate with each other through synaptic connections, creating an intricate network.
Studying single neurons has been crucial in understanding the fundamental principles of neural communication. By observing and measuring the behavior of individual neurons, researchers have been able to uncover key insights, giving us a foundation to build upon.
The Emergence of Mathematical Modeling in Neuroscience
As our understanding of single neurons grew, researchers sought to tackle more complex phenomena, such as how groups of neurons interact to produce specific behaviors or cognitive processes. This is where mathematical modeling stepped in as a powerful tool.
Mathematical models allow scientists to simulate and predict the behavior of neural systems, providing valuable insights that can be tested and refined over time. These models provide a bridge between theory and experimentation, bringing us closer to understanding the brain's complexities.
From Single Neurons to Neural Fields: The Power of Scale
While single neurons provide valuable information, it is their collective behavior that shapes our thoughts and perceptions. Neural fields take into account the interactions between large populations of neurons, allowing us to study higher-level phenomena.
By modeling neural fields, researchers can explore how complex patterns and dynamics emerge from the collective behavior of neurons. These models reveal important principles, such as synchronization, oscillations, and spatial patterns, shedding light on various brain functions like vision, memory, and decision-making.
Real-World Applications: The Impact of Mathematical Modeling
The impact of mathematical modeling in neuroscience reaches far beyond theoretical understanding. These models have practical applications in numerous fields, including medicine, robotics, and artificial intelligence.
For instance, understanding how neural networks operate has helped in developing more advanced brain-computer interfaces, enabling paralyzed individuals to control prosthetic limbs through their thoughts. Similarly, modeling neural fields has contributed to advancements in computer vision and image recognition algorithms.
Challenges and Future Directions
While mathematical modeling has undoubtedly revolutionized neuroscience, many challenges still lie ahead. The brain is an incredibly complex organ, and fully capturing its intricacies remains a daunting task. Researchers continue to refine existing models and develop new approaches to tackle unsolved mysteries.
The future of mathematical modeling in neuroscience is promising. As computational power increases and new technologies emerge, scientists will be able to build more accurate and comprehensive models. This, in turn, will lead to breakthroughs in understanding brain disorders, developing more effective treatments, and creating advanced artificial intelligence systems.
The journey from single neurons to neural fields is a captivating one. By using mathematical modeling, scientists have unlocked fascinating insights into how our brains function. These models have revolutionized our understanding of neural communication and have real-world applications that impact our lives in profound ways.
As we continue to explore the wonders of the brain, mathematical modeling will continue to be a vital tool in unraveling its complexities. The journey from understanding single neurons to comprehending neural fields opens up exciting possibilities and brings us one step closer to unlocking the secrets of the mind.
4 out of 5
| Language | : | English |
| File size | : | 965 KB |
| Text-to-Speech | : | Enabled |
| Screen Reader | : | Supported |
| Enhanced typesetting | : | Enabled |
| Word Wise | : | Enabled |
| Print length | : | 67 pages |
| Lending | : | Enabled |
Waves in Neural Media: From Single Neurons to Neural Fields surveys mathematical models of traveling waves in the brain, ranging from intracellular waves in single neurons to waves of activity in large-scale brain networks. The work provides a pedagogical account of analytical methods for finding traveling wave solutions of the variety of nonlinear differential equations that arise in such models. These include regular and singular perturbation methods, weakly nonlinear analysis, Evans functions and wave stability, homogenization theory and averaging, and stochastic processes. Also covered in the text are exact methods of solution where applicable. Historically speaking, the propagation of action potentials has inspired new mathematics, particularly with regard to the PDE theory of waves in excitable media. More recently, continuum neural field models of large-scale brain networks have generated a new set of interesting mathematical questions with regard to the solution of nonlocal integro-differential equations.
Advanced graduates, postdoctoral researchers and faculty working in mathematical biology, theoretical neuroscience, or applied nonlinear dynamics will find this book to be a valuable resource. The main prerequisites are an introductory graduate course on ordinary differential equations or partial differential equations, making this an accessible and unique contribution to the field of mathematical biology.

 Anthony Burgess
Anthony BurgessEverything You Need To Know About Building Referral...
Are you looking for ways to boost revenue...

 Aleksandr Pushkin
Aleksandr PushkinThe Fascinating History of Afro Uruguay - Unveiling the...
Afro Uruguay refers to the rich and diverse...

 Anton Foster
Anton FosterReflections From Stubborn Son: A Journey of...
Have you ever encountered a stubborn...

 Brennan Blair
Brennan BlairDiscover the Revolutionary World of Protein Modelling:...
Protein modelling is an essential...

 Ricky Bell
Ricky BellThe Best Old Fashioned Advice: Timeless Wisdom Passed...
Have you ever turned to your grandparents,...

 Isaiah Price
Isaiah PriceEmbark on an Unforgettable Journey: The Sword and Sorcery...
Are you ready to be...

 Hassan Cox
Hassan CoxThe Enchanting World of Wendy Darling Comes Alive in...
Step into the magical world of Neverland...

 Ivan Turner
Ivan TurnerAdsorption Calculations And Modelling Chi Tien: Unlocking...
In the field of chemistry, adsorption is a...

 Harvey Hughes
Harvey HughesUnleashing the Full Potential of a Team: How To Organize...
"Genius is 1% inspiration and 99%...

 Desmond Foster
Desmond FosterThe Fascinating Journey of George Romanes: From...
George John Romanes, born on May 20, 1848,...

 Adrien Blair
Adrien BlairThe Untold Truth: The Bible In The Early Church - A...
Lorem ipsum dolor sit amet, consectetur...
Light bulbAdvertise smarter! Our strategic ad space ensures maximum exposure. Reserve your spot today!

 Fernando PessoaThe Ultimate Poultry Practical Guide: A Comprehensive Manual for Breeding,...
Fernando PessoaThe Ultimate Poultry Practical Guide: A Comprehensive Manual for Breeding,... Victor TurnerFollow ·13.3k
Victor TurnerFollow ·13.3k Hugo CoxFollow ·5k
Hugo CoxFollow ·5k Isaiah PowellFollow ·13.5k
Isaiah PowellFollow ·13.5k Isaac AsimovFollow ·4.5k
Isaac AsimovFollow ·4.5k Ernest HemingwayFollow ·12.3k
Ernest HemingwayFollow ·12.3k Mark MitchellFollow ·12.6k
Mark MitchellFollow ·12.6k Derek CookFollow ·12.6k
Derek CookFollow ·12.6k Pat MitchellFollow ·14.6k
Pat MitchellFollow ·14.6k






















